How do we put into words what we mean by a “square”? Here are six naughty shapes pretending to be squares. But they are all fakes! Can you see what’s wrong with each bad definition?
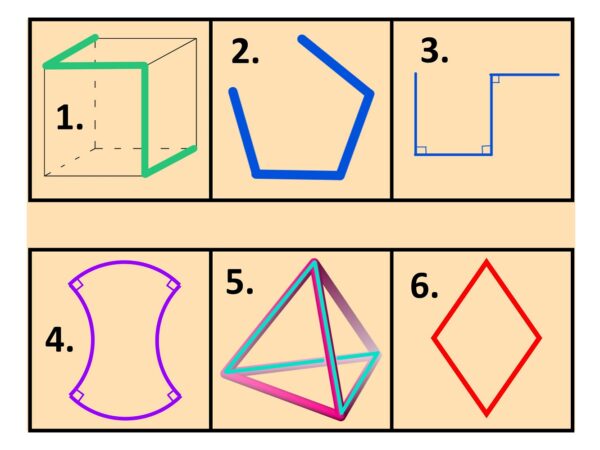
1) SQUARE: A SHAPE WITH FOUR SIDES OF EQUAL LENGTH: but hang on – this shape is not flat! It just goes around four edges of a cube. Hmm, let’s try another definition:
Read the rest of this page »
NUMBERS: are an abstract idea used to measure e.g. how many? How big? Or numbers can point to a position on a number line. Here are some examples of numbers: $4, \frac{3}{8}, 0.157, \pi, -\sqrt{2}, $ fifty-nine. There are infinitely many different numbers.
NUMERALS: are the words or symbols that we use the represent our numbers. The list above is really a list of numerals. I could represent the number 4 using a numeral or by clapping my hands four times, or by holding up four fingers.
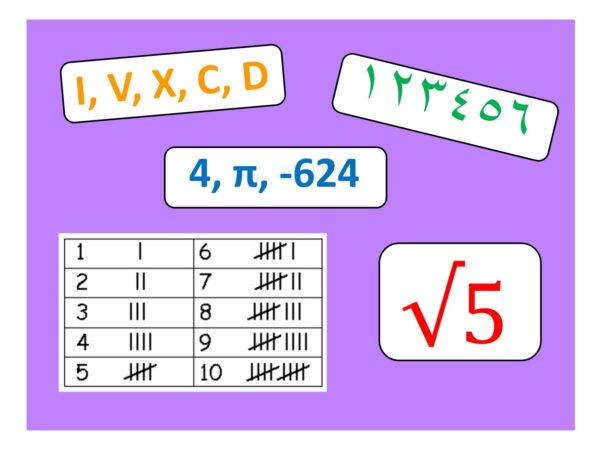
DIGITS: are the ten single symbols 0, 1, 2, 3, 4, 5, 6, 7, 8, 9 that we use to represent numbers.
Read the rest of this page »
Your challenge today is to arrange the numbers 1 to 9 in a row so that each pair of adjacent digits appears as an answer in the 10 by 10 times table square.
ER… Shall I run you past that again??
To illustrate what this means, let’s first try just putting the numbers down in numerical order:
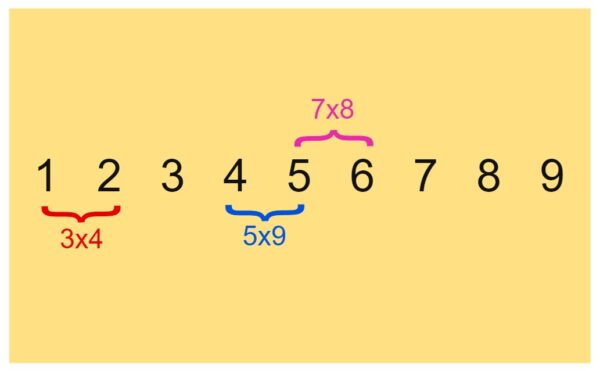
As you can see, three of the adjacent pairs appear as answers in the first ten times tables:
Read the rest of this page »
COUNTING CANNONBALLS
This pyramid of cannonballs appears at the foot of the Armada Monument on Plymouth Hoe. It consists of six layers: a single cannonball at the apex, resting on a square of 4 cannonballs, resting on a square of 9 cannonballs, and so on for a total of 6 layers.

A reasonably curious mathematician might wonder how many cannonballs there are in the entire pyramid (including the “hidden” ones you can’t see on the inside of the pyramid).
The answer is the sixth Square Pyramidal number – which I’ll call $P_6$
THE-NOT-VERY-CLEVER-WAY:
To find the total we could just go from layer to layer, adding as we go:
Read the rest of this page »
INTRODUCING $\pi$: IT’S AS EASY AS PI:
Circles are very special – more so than rectangles. Rectangles, you see, come in many different sizes (big, small, enormous etc) and also in many different shapes (tall and thin, short and stubby, square etc).
Circles also come in many different sizes (big, small, enormous etc.) but unlike rectangles, all circles are exactly the same shape. That’s right: even if two circles have different circumference (the distance all the way around the outside) and different diameter (the distance across the middle from one side to the other) the two will always be in the same ratio. This ratio of C/D (circumference divided by diameter) always gives the number $\pi$ (pronounced “pi” – but nothing to do with the pie that you eat with chips, peas and gravy).
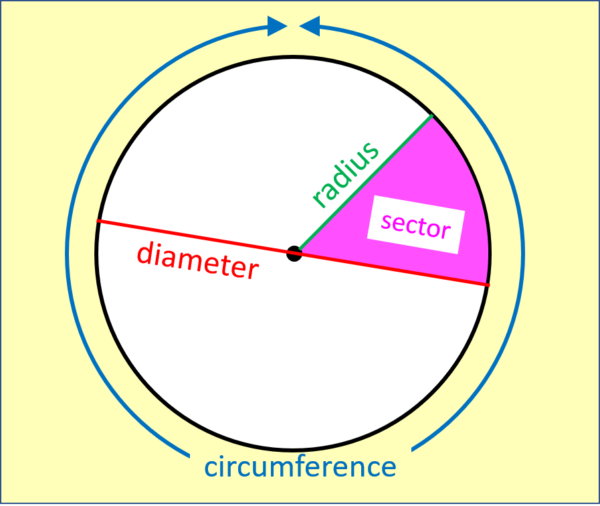
CAN I TRY?
Read the rest of this page »

This sequence – the “Dudeney numbers” – lists all the numbers equal to the digit sum of their cubes.
ER, WHAT NOW??
For instance, 17 is on the list because when you cube it (17x17x17 = 4913) and then take the digit sum (4+9+1+3 = 17) you get back to where you started. Neat!
Read the rest of this page »
TIMES TABLE SECRETS, CUBE NETS, OCTAHEDRA, PALINDROMES AND ALTERNATING DIGIT SUMS: LET’S HEAR IT FOR NUMBER ELEVEN!
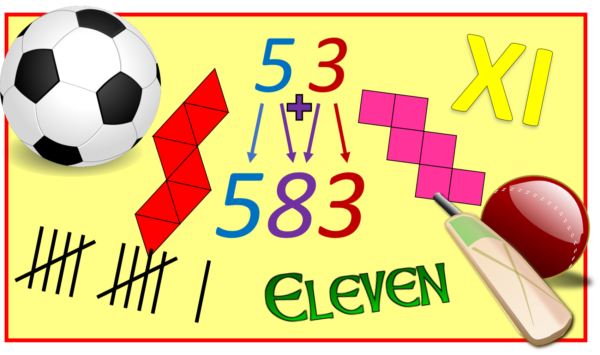
NETFLIX: the Producers of instant cult TV series “Stranger Things” clearly appreciate the wonder of the number Eleven: they even (or should that be “oddly”) named the series’ main character “Eleven”.
CUBE NETS: there are eleven distinct nets of cubes.
Read the rest of this page »
To celebrate the traditional gym membership peak associated with the New Year, here are some muscles whose names have mathematical associations. As you read, see if you can match the muscle to the image in this picture:
SCALENE: a set of three (or occasionally four) muscles in each side of the neck, so named as they are all of different lengths.
Read the rest of this page »
COUNTING AND SYMMETRY GAMES FOR RECEPTION TO YEAR 6
THE “WHAT” GAMES?? the strips of metal joining the hub (centre) of a wheel to its rim (edge) are called the spokes. Have you tried to count the number of spokes on a car wheel? How good is your counting? What other patterns can you find in the wheels?
SAFETY RULES:
- Do not attempt to count the spokes on a moving car. Only count the spokes if it is parked.
- Small children should hold an adult’s hands.
- Ask permission if there is someone sat inside the car you want to count. Explain that you are playing a maths game and that their car has really cool wheels!
GAME 1- COUNT THE SPOKES: Some car wheels have 5 spokes, some have 7, some have more. Find some cars and count the number of spokes.
GAME 2– COLLECT THE SET: how many different numbers can you find? Here are wheels with every number of spokes from 4 up to 16. I can’t find 17!! – can you?

Read the rest of this page »
WHERE MATHS MEETS GEOGRAPHY
1) COUNTING SHEEP: Wales is famous for its sheep, but are there more sheep or more people in Wales? Have a guess, answer later on!

2) VISIT THE STEEPEST STREET IN THE WORLD!! with a calf-busting gradient of
Read the rest of this page »









